What is the triangle method? This method, a powerful problem-solving tool, is surprisingly versatile. It’s not just about geometry; it’s a structured approach that can help you tackle complex issues across various fields. From project management to personal decision-making, understanding the triangle method’s principles and components unlocks a new level of clarity and efficiency.
This guide dives deep into the core concepts of the triangle method, exploring its historical context, components, applications, procedures, tools, and even comparisons with other problem-solving approaches. We’ll provide clear examples and practical scenarios to illustrate its application.
Introduction to the Triangle Method
The triangle method, a powerful tool in various fields, provides a structured approach to problem-solving. It involves constructing a triangle, with each side representing a key component or factor. This method simplifies complex issues by visually representing the relationships between these components. Understanding the triangle method allows for a clearer comprehension of interconnected elements and their impact on the overall outcome.The fundamental principles behind the triangle method rely on the concept of interdependency.
It assumes that factors are interconnected and influence each other. This approach helps to identify critical elements, understand their relationships, and ultimately develop effective solutions. The visualization provided by the triangle framework helps in analyzing the situation from different perspectives, facilitating a holistic view.
Common Use Cases
The triangle method finds applications in diverse fields. Its structured nature makes it valuable for decision-making processes, project planning, and risk assessment. By visually representing the key components and their interactions, the method facilitates a comprehensive understanding of the problem or situation.
- Project Management: In project management, the triangle method can be used to analyze the trade-offs between time, cost, and scope. A triangle can visually represent these three elements, demonstrating how changes in one can impact the others. This helps in making informed decisions regarding resource allocation and scheduling.
- Engineering Design: Engineers utilize the triangle method to analyze constraints and trade-offs during the design process. For instance, a triangle can represent the relationship between material strength, weight, and cost, helping engineers make optimal design choices.
- Financial Planning: Individuals and businesses use the triangle method to assess the interplay between investment strategies, risk tolerance, and potential return. This allows for a better understanding of the trade-offs involved in various investment options.
Historical Context
While there isn’t a specific, documented historical context for the “triangle method” as a formally named technique, the underlying principles of visually representing interconnected factors have been present in various fields for centuries. Early forms of this approach can be observed in ancient Greek geometrical problem-solving, which relied on visual representations to understand and solve mathematical puzzles.
Examples of Application
Consider a project manager planning a construction project. Time, cost, and scope are represented by the three sides of a triangle. If a change in the project’s scope requires additional resources, the triangle can visually illustrate how this increase in scope impacts both time and cost. Another example is an engineer designing a bridge. The triangle method allows the engineer to consider the interplay between the material’s strength, the bridge’s weight, and its overall cost.
These examples showcase how the triangle method provides a structured approach to problem-solving, highlighting the interconnectedness of factors.
Components of the Triangle Method
The Triangle Method, a powerful tool for visualizing and analyzing relationships, presents information in a structured triangular format. Understanding its components is crucial for effectively interpreting the data and drawing meaningful conclusions. This method, often employed in various fields from business strategy to scientific research, emphasizes the interconnectedness of different elements, allowing for a holistic view of a particular system or process.The triangle itself is not merely a geometric shape; it represents a dynamic interplay between different facets of a problem or concept.
Each corner and side of the triangle represents a key component, and their relative position and length often indicate the strength of the relationship between them. Analyzing these components and their interplay is essential to understanding the underlying mechanisms and driving forces at play.
Key Elements of the Triangle
The Triangle Method relies on three primary components that form the vertices of the triangle. Each component holds a unique significance and contributes to the overall understanding of the system being analyzed. Their relationships determine the shape and the implications of the triangle.
| Component | Description | Example | Importance |
|---|---|---|---|
| Base | The base of the triangle represents the fundamental principle or the core issue at hand. It provides the foundation for the entire analysis. | In a business strategy, the base might be the overall market demand for a particular product. | The base anchors the analysis and dictates the direction of the other elements. Without a clear base, the entire triangle loses its meaning. |
| Apex | The apex of the triangle represents the ultimate goal, objective, or desired outcome. It signifies the target or destination. | In a marketing campaign, the apex might be achieving a 20% increase in sales. | The apex motivates the analysis and provides a clear direction for achieving the desired results. |
| Side 1 (connecting Base to Apex) | This side represents the primary means or strategy to achieve the desired outcome (apex) based on the fundamental principle (base). | In a product development project, the strategy could be focused on using a new technology to meet consumer demand. | This side defines the specific path or action plan. The effectiveness of this side directly impacts the overall success of the triangle. |
| Side 2 (connecting Base to Apex) | This side represents a secondary or alternative strategy to achieve the desired outcome (apex) based on the fundamental principle (base). | Using social media marketing alongside traditional marketing channels. | It provides a backup plan or a complementary approach. Having a backup plan enhances the robustness of the triangle. |
Interrelationships Within the Triangle, What is the triangle method
The relationships between the base, apex, and sides are crucial to understanding the dynamics of the Triangle Method. The length and position of each side, relative to the base and apex, signify the strength and importance of the corresponding strategies. A longer side suggests a more significant role in achieving the desired outcome. The angle between the sides influences the potential synergy or conflict between the strategies.
Analyzing these interrelationships is key to maximizing effectiveness.
Applications and Variations
The triangle method, a powerful tool for analyzing relationships between variables, finds diverse applications across various disciplines. Its flexibility allows for adaptations to suit specific needs, making it a valuable tool for problem-solving and decision-making. This section delves into practical applications, variations, and the strengths and limitations of using the triangle method in different contexts.The core principle of the triangle method—visualizing and quantifying the interdependencies among factors—is remarkably adaptable.
It can be employed in scenarios ranging from project management to financial analysis and even social sciences. By understanding the strengths and limitations of each application, we can effectively leverage the method for improved outcomes.
Applications in Project Management
The triangle method is frequently used in project management to estimate project durations and costs. It visualizes the relationship between task durations, budget constraints, and project timelines. This visual representation allows project managers to identify potential risks and make informed decisions regarding resource allocation and scheduling. For example, a project manager might use a triangle method to depict the possible project completion dates based on different levels of budget allocation and the varying duration of different tasks within the project.
Applications in Financial Analysis
In financial analysis, the triangle method can be applied to assess investment risks and returns. This application visualizes the relationship between investment capital, potential returns, and associated risks. By plotting these variables on a triangle, analysts can identify potential investment opportunities and make informed decisions regarding portfolio allocation. For instance, an investment advisor might use a triangle method to illustrate the trade-offs between potential return and risk level across different investment options.
Applications in Social Sciences
The triangle method can also be utilized in social sciences to analyze complex social phenomena. For example, it can be applied to examine the interrelationships between social structures, individual behaviors, and societal outcomes. Researchers can utilize this method to visualize the interactions and dependencies among various factors affecting a specific societal issue.
Variations of the Triangle Method
The triangle method is not confined to a single, rigid format. Various adaptations exist to suit the specific needs of different fields. For example, in project management, a weighted triangle method can be used to assign different weights to various factors in the analysis. In social sciences, a more complex, multi-dimensional triangle method could be utilized to analyze the interrelationships among multiple variables.
Strengths and Limitations
The triangle method offers several strengths, including its visual nature, which makes it easy to understand and interpret complex relationships. However, its limitations stem from the simplification of complex issues into a three-dimensional model.
Comparison Table
| Field | Application | Description | Example |
|---|---|---|---|
| Project Management | Estimating Project Durations | Visualizing the relationship between task durations, budget, and project timeline. | Analyzing the impact of budget cuts on project completion dates. |
| Financial Analysis | Assessing Investment Risks | Visualizing the relationship between investment capital, potential returns, and associated risks. | Identifying investment options with acceptable risk levels and potential returns. |
| Social Sciences | Analyzing Social Phenomena | Visualizing the interrelationships between social structures, individual behaviors, and societal outcomes. | Examining the factors contributing to poverty rates in a specific region. |
Procedures and Steps
The triangle method, a powerful tool for solving various geometric and practical problems, offers a structured approach. Understanding its procedures is key to successfully applying the method in diverse situations. This section details the step-by-step procedure, providing clear instructions and examples to enhance comprehension.The triangle method’s efficacy lies in its methodical approach. Each step builds upon the previous one, ensuring a logical progression toward the desired solution.
By following these procedures, users can confidently tackle problems, from simple calculations to complex applications.
Step-by-Step Procedure
The triangle method’s efficiency stems from its straightforward procedure. Following these steps will guide users through the process, ensuring accurate results.
- Problem Definition and Analysis: Clearly define the problem and identify the known and unknown quantities. Sketch a diagram if applicable, labeling relevant sides and angles. This initial step is crucial for understanding the problem’s context and determining the appropriate triangle method application.
- Identify the Relevant Triangle: Determine which triangle (or triangles) within the given figure or scenario is relevant to the problem. Highlight or label the triangle to focus attention and avoid confusion.
- Identify Known Values: Carefully list all known values, including side lengths, angles, and any other given information. Ensure accurate measurements and data entry.
- Select the Appropriate Formula: Choose the trigonometric function or formula applicable to the specific problem. This decision depends on the known values and the desired result. Common formulas include sine, cosine, tangent, or the Pythagorean theorem, depending on the problem.
- Substitution and Calculation: Substitute the known values into the chosen formula. Perform the calculations accurately. Use a calculator to find the unknown value.
- Verification and Interpretation: Review the calculations and check for errors. Ensure the result aligns with the problem’s context and expectations. Interpret the results in the context of the problem, ensuring a complete and accurate solution.
Example Application
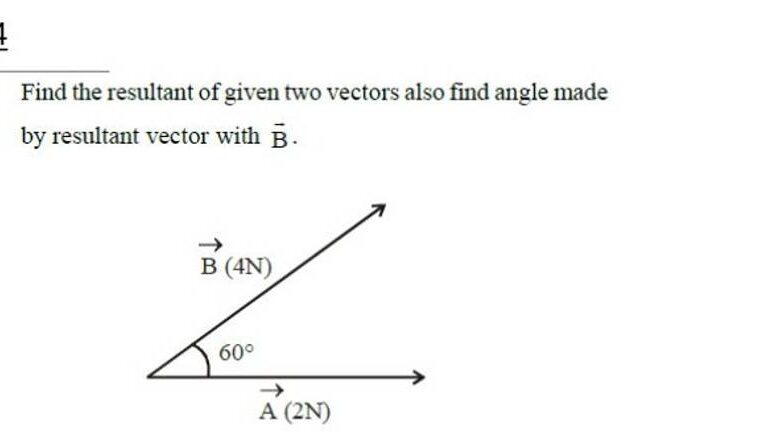
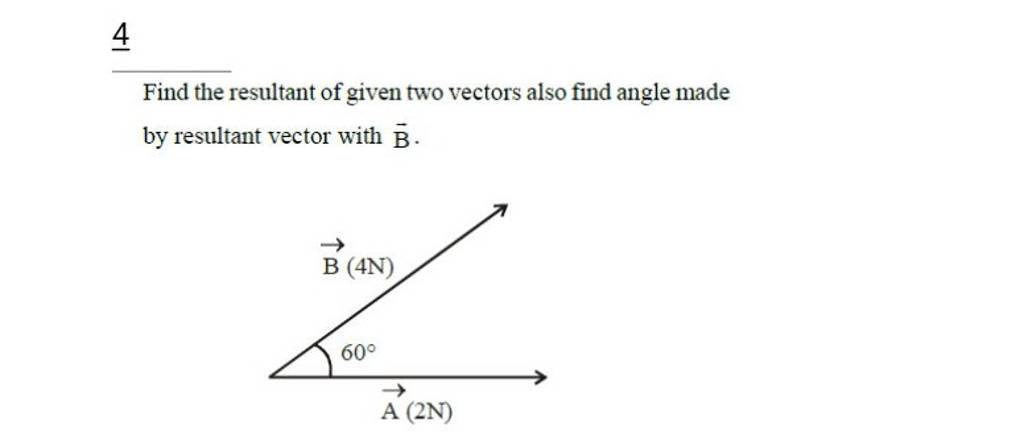
The triangle method is useful in various practical situations. Consider finding the height of a building.
Scenario: A person stands 50 meters away from a building. The angle of elevation to the top of the building is 30 degrees. Determine the height of the building.
- Problem Definition: Determine the height of the building.
- Relevant Triangle: A right-angled triangle is formed with the person, the base of the building, and the top of the building. The height of the building is the unknown side.
- Known Values: Distance from person to building (adjacent side) = 50 meters, Angle of elevation = 30 degrees.
- Appropriate Formula: Tangent function (tan θ = opposite/adjacent).
- Substitution and Calculation: tan(30°) = height/
50. Solving for height
height = 50
tan(30°) ≈ 28.87 meters.
- Verification and Interpretation: The calculated height of the building is reasonable in the context of the problem.
Tools and Techniques
The triangle method, while conceptually straightforward, benefits significantly from the use of appropriate tools and techniques. These tools streamline the process, improve accuracy, and enable more comprehensive analysis. Choosing the right tools depends on the specific context and the desired level of detail. Effective utilization of these tools can drastically enhance the overall effectiveness of the method.
Spreadsheet Software
Spreadsheet software, such as Microsoft Excel or Google Sheets, is invaluable for organizing and manipulating data in the triangle method. Data entry, calculation of areas, and visualization of results are significantly simplified. The ability to create charts and graphs further enhances the presentation and interpretation of findings. The use of formulas for automatic calculations, particularly for areas and perimeters, is a powerful asset.
Geometric Drawing Software
Geometric drawing software, like GeoGebra or AutoCAD, provides a visual representation of the triangles involved. This visual component facilitates a deeper understanding of the relationships between the sides and angles of the triangles. The ability to precisely draw and measure shapes is crucial for accuracy. This software also aids in exploring different triangle configurations and their implications.
Calculators
Calculators, both physical and digital, are essential tools for performing calculations. Basic calculators are sufficient for simple calculations, while scientific calculators are useful for more complex calculations involving trigonometric functions and other mathematical operations. The use of calculators ensures accuracy in measurements and reduces the risk of errors, crucial for obtaining reliable results.
Data Visualization Tools
Tools like Tableau or Power BI allow for the creation of interactive visualizations of the triangle method data. This visualization aids in identifying patterns, trends, and insights that might be missed in raw data. Interactive dashboards enable real-time monitoring and analysis of data changes. These tools can provide compelling visuals that are essential for effective communication and presentations.
| Tool/Technique | Description | Function | Example |
|---|---|---|---|
| Spreadsheet Software | Software for organizing, manipulating, and calculating data. | Data entry, calculations (area, perimeter), visualization (charts, graphs). | Using Excel to input triangle side lengths, calculate areas, and generate a chart comparing different triangle types. |
| Geometric Drawing Software | Software for creating and manipulating geometric shapes. | Precise drawing of triangles, measurement of sides and angles, exploring different configurations. | Using GeoGebra to construct a right-angled triangle and measure its angles and sides. |
| Calculators | Devices for performing mathematical calculations. | Performing calculations involving trigonometric functions, areas, and perimeters. | Using a scientific calculator to compute the sine, cosine, or tangent of an angle in a triangle. |
| Data Visualization Tools | Software for creating interactive visual representations of data. | Identifying patterns, trends, and insights in data; real-time monitoring and analysis. | Creating a dashboard in Tableau to track changes in triangle area based on varying side lengths. |
Illustrative Examples: What Is The Triangle Method
The Triangle Method, a powerful tool for analyzing and optimizing complex systems, finds applications in various fields. Understanding its practical use requires concrete examples. This section provides illustrative cases and scenarios to demonstrate the method’s effectiveness. From project management to engineering design, the Triangle Method offers a structured approach to planning and execution.The examples below showcase how the Triangle Method can be used to estimate project timelines, resource allocation, and potential risks.
Each example includes a narrative description, a visual representation (a diagram), and a breakdown of the key components of the Triangle Method applied in that context.
Detailed Example: Software Development Project
This example illustrates the Triangle Method’s application in estimating the time needed for a software development project.
A software company, “InnovateTech,” is developing a new mobile application. The project manager, Sarah, wants to estimate the project timeline. Using the Triangle Method, she considers three scenarios: optimistic, pessimistic, and most likely. The optimistic scenario assumes everything goes smoothly, the pessimistic scenario anticipates potential roadblocks, and the most likely scenario represents a realistic projection.
Optimistic estimate: 3 months
Pessimistic estimate: 6 months
Most likely estimate: 4 months
The project’s estimated timeline is represented by the triangle formed by these three points. The most likely estimate of 4 months forms the base of the triangle, while the optimistic and pessimistic estimates (3 and 6 months, respectively) form the other two points. This visual representation allows for a clear understanding of the potential range of project completion dates.
| Estimate Type | Time (Months) |
|---|---|
| Optimistic | 3 |
| Most Likely | 4 |
| Pessimistic | 6 |
The project manager can now use this estimated range to create a more realistic project plan, accounting for potential delays and uncertainties. The visual representation provides a clear picture of the project’s possible duration, enabling better risk management and resource allocation.
Practical Case Study: Construction Project
Consider a construction project for a new office building. The project involves several phases, including foundation laying, structural work, and interior finishing. Applying the Triangle Method, the project manager estimates each phase’s duration using the optimistic, pessimistic, and most likely estimates.
Using the Triangle Method, a construction project manager can estimate the time needed for various phases. The method helps visualize the project’s timeline and potential delays. By considering the optimistic, pessimistic, and most likely estimates for each phase, the project manager can determine a range of possible completion times. This enables better planning and resource allocation.
The diagram below visualizes the timeline estimation for the construction phases.
(Diagram) A triangle is drawn with the base representing the most likely duration of each phase, with the left and right sides representing optimistic and pessimistic durations, respectively. The entire project duration is shown by connecting the tops of these triangles.
Multiple Examples: Scenario Illustrations
The Triangle Method can be applied to diverse scenarios. These include estimating the time needed for a marketing campaign, the cost of manufacturing a product, or the number of resources required for a project. In each case, the Triangle Method provides a framework for estimating parameters under varying conditions.
- Marketing Campaign: Estimating the time to reach a specific target audience using the Triangle Method, the estimated time range is between 2 to 4 months, with a most likely duration of 3 months. This gives a realistic picture of the campaign’s potential duration.
- Product Manufacturing: The estimated cost of manufacturing a product can be determined using the Triangle Method. The method estimates the cost under optimistic, pessimistic, and most likely scenarios.
- Project Resource Allocation: The Triangle Method aids in determining the number of resources needed for a project. The method uses optimistic, pessimistic, and most likely scenarios to estimate the number of resources required.
Comparison with Other Methods
The triangle method, while effective for certain surveying and engineering tasks, isn’t the only approach available. Understanding its strengths and weaknesses relative to other methods allows for informed decision-making in choosing the most appropriate technique for a given project. This comparison highlights key distinctions, enabling users to tailor their approach to specific needs and constraints.Comparing the triangle method with alternative approaches reveals crucial differences in their underlying principles and practical applications.
A systematic evaluation considers factors like accuracy, efficiency, and the nature of the data required. Analyzing the strengths and weaknesses of each method helps to determine the best fit for particular situations.
Comparison Table
The table below provides a structured comparison of the triangle method with other commonly used surveying techniques.
| Method | Key Characteristics | Strengths | Weaknesses |
|---|---|---|---|
| Triangle Method | Based on the principle of trigonometry, utilizing known angles and distances to calculate unknown lengths and positions. Typically involves establishing a network of triangles. | Generally accurate for large-scale projects, especially in terrain with limited access. Provides good control points for subsequent surveying work. Relatively straightforward to implement for simple cases. | Can be time-consuming for complex projects involving numerous triangles. Susceptible to errors in angle measurement, particularly in challenging terrain. Requires precise instrument calibration and skilled operators. |
| Traverse Surveying | A linear method, measuring angles and distances along a series of connected lines. | Suitable for smaller areas and detailed mapping. Relatively easy to implement, requiring fewer instruments compared to triangulation. Provides a clear record of linear measurements. | Accuracy depends on the length of the traverse. Accumulation of errors along the traverse can affect overall accuracy. Less suitable for large-scale projects. |
| GPS Surveying | Utilizes satellite signals to determine the precise coordinates of points. | Extremely fast and efficient for large-scale projects. Provides high accuracy, especially in open areas. Minimizes the need for manual measurements. | Reliance on satellite signals can be affected by atmospheric conditions. Can be expensive to implement, requiring specialized equipment. Signal interference can affect measurements. |
| Total Station Surveying | Combines electronic distance measurement (EDM) with theodolites to measure angles and distances simultaneously. | Offers high accuracy in both angle and distance measurements. Fast and efficient, reducing the time required for manual measurements. Provides data in digital format. | Cost of equipment can be significant. Requires skilled operators for optimal use. Instrument malfunction can lead to errors. |
Factors Affecting Choice
Several factors influence the choice between the triangle method and other surveying techniques. Project scale, terrain characteristics, budget constraints, and desired accuracy are crucial considerations. For instance, a large-scale land surveying project in rugged terrain might favor the triangle method or GPS, while a detailed survey of a small plot of land might benefit from traverse surveying. The available resources and expertise also play a significant role in the decision-making process.
Strengths and Weaknesses Summary
The triangle method, while robust for large-scale projects in challenging terrain, has limitations in terms of time and complexity. Other methods, such as traverse surveying, offer simplicity and speed for smaller projects. GPS and total station surveying provide high accuracy and efficiency but can be more expensive and require specialized equipment. Careful consideration of these strengths and weaknesses, coupled with an understanding of project specifics, allows for the optimal selection of the most appropriate surveying technique.
Ultimate Conclusion
In conclusion, the triangle method, despite its simplicity, offers a structured and effective way to approach problems. By understanding its key components, procedures, and potential applications, you can harness its power to make informed decisions and achieve your goals more efficiently. We hope this exploration of the triangle method has provided valuable insights and practical tools for your own problem-solving endeavors.
